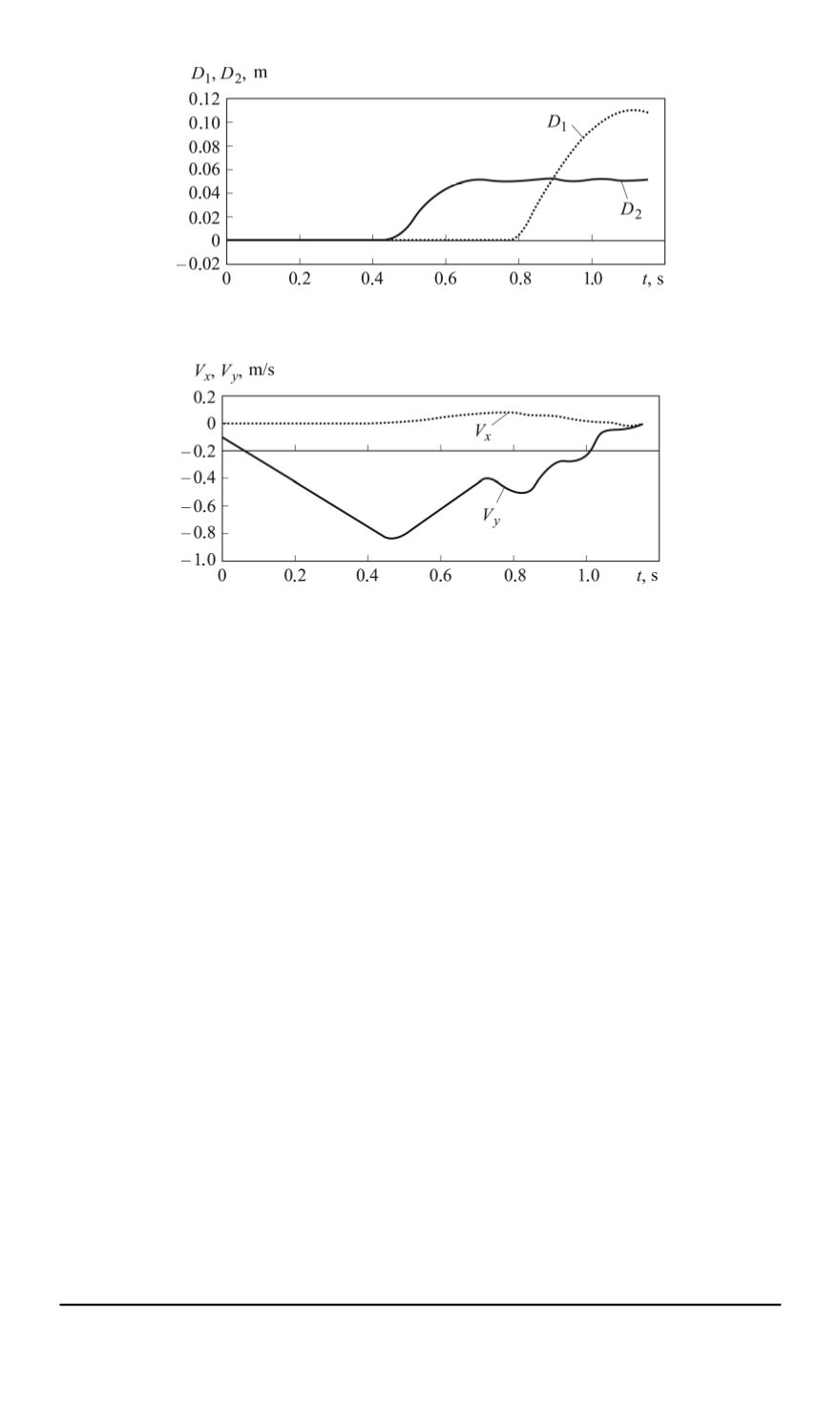
Fig. 7. Graphs of variance of the deformations
D
1
и
D
2
of the shock absorbers of
the first and the second legs of the spacecraft
Fig. 8. Graphs of variance of velocities
V
x
and
V
y
during the spacecraft landing
the first leg has the largest deformation. The strut inclination angle of the
first leg is
α
f
= 33
.
4
◦
after landing.
Fig. 8 shows graphs of variance of the velocities
V
x
and
V
y
during
the spacecraft landing. It is evident that during the contact of the second
and the third legs with the soil, the velocity
V
x
starts increasing due to a
length change of their shock absorbers, while the magnitude of
V
y
starts
decreasing. At the end of the spacecraft landing, these velocities are equal
to zero.
Let us note that after the complete landing, the spacecraft inclination
angle relative to the surface of the Moon is
ϑ
f
=
−
2
.
87
◦
.
Conclusions.
1. The article describes a method for estimating some
longitudinal motion parameters of a spacecraft during landing on the surface
of a small celestial body. The method considers the reaction applied to the
movable legs from the surface soil and operation of the thrusters.
2. The authors use the spacecraft landing on the surface of the Moon
as an example for estimating the impact of excitations on both the legs
position change and forces in the shock absorbers. The authors also consider
safe landing conditions: in case of a spacecraft vertical landing, the shock
absorber force has a value which allows getting reasonable angles of the
struts at moderate soil reaction forces on the legs after landing. The authors
discuss the impact of the spacecraft initial inclination relative to the Moon’s
surface on the dynamics of its motion during landing. The analysis reveals
ISSN 0236-3941. HERALD of the BMSTU. Series “Mechanical Engineering”. 2014. No. 1 33