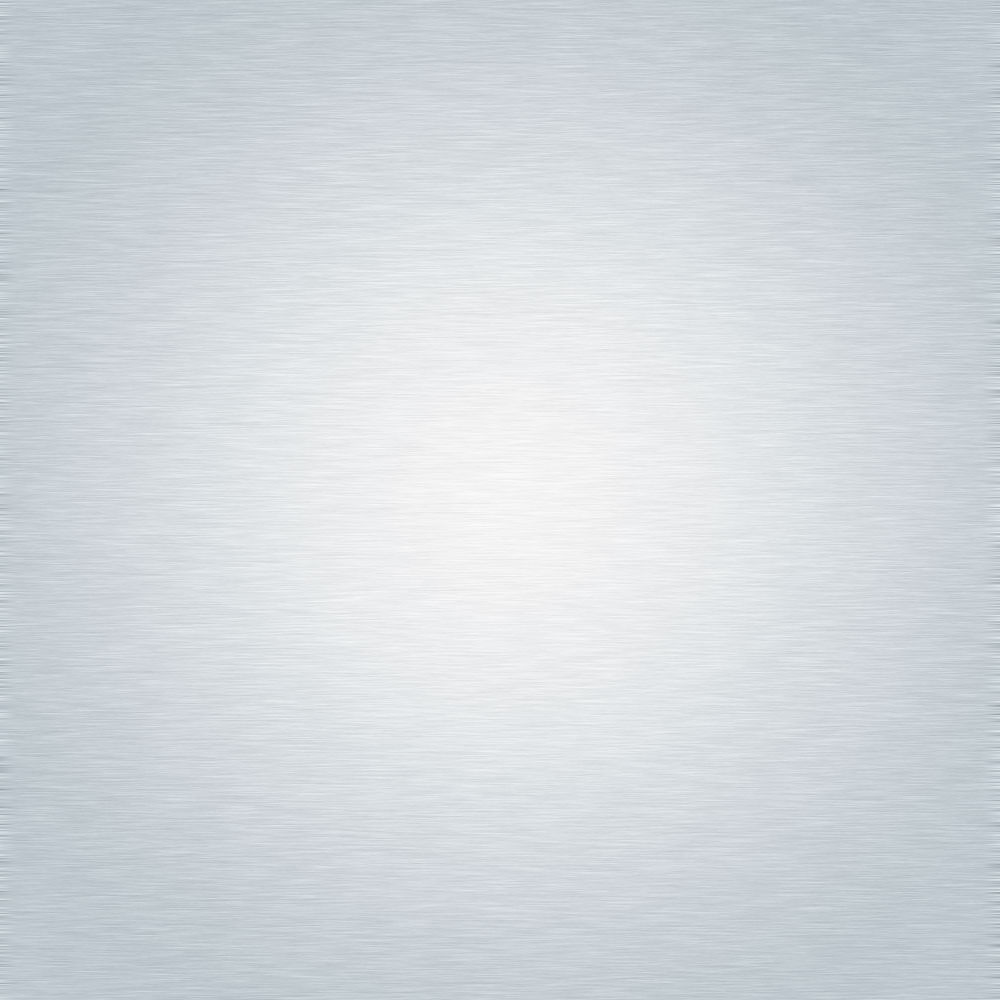
dV
x
dt
=
1
m
[
F
T
1
+
F
T
2
X
+
F
T
3
X
−
(
P
1
+
P
2
+
P
3
) sin
ϑ
]
−
g
sin
θ
g
;
dV
y
dt
=
1
m
[
F
N
1
+
F
N
2
+
F
N
3
+ (
P
1
+
P
2
+
P
3
) cos
ϑ
]
−
g
cos
θ
g
;
dx
dt
=
V
x
;
dy
dt
=
V
y
;
dω
z
dt
=
1
I
z
[(
F
T
1
+
F
T
2
X
+
F
T
3
X
)
y
+
F
N
1
x
1
−
−
(
F
N
2
+
F
N
3
)
x
2
+
P
1
x
p
−
P
2
x
p
cos
γ
2
−
P
3
x
p
cos
γ
3
];
dϑ
dt
=
ω
z
.
(1)
The legs dimensions
h
0
,
h
1
,
H
,
l
E
,
l
0
— are selected beforehand. The
initial values of the following parameters are determined by the formulae:
α
0
= arcsin
H
−
h
0
l
0
;
l
sw0
=
p
(
l
0
cos
α
0
)
2
+ (
H
+
h
1
)
2
;
β
0
= arcsin
l
0
cos
α
0
l
sw0
.
(2)
Let us consider a solution algorithm for the problem of the spacecraft
landing on the surface of a celestial body for the first (second) leg, which
are directed along the
OX
axis of the SCS.
The contact condition between the first (second) leg and the surface can
be written as follows:
δy
1
=
y
−
y
1
= 0
,
(3)
here
y
1(2)
=
h
0
cos
ϑ
−
l
E
sin
ϑ
+
l
0
(
α
0
−
ϑ
1(2)
);
(4)
ϑ
2
= arcsin(cos(
π
−
γ
2
) sin
ϑ
)
(5)
— is an inclination angle of the spacecraft for the second leg.
For
δy
1(2)
= 0
we find the current values for
y
and
ϑ
, which are
determined during the integration of equations (1):
α
1(2)
= arcsin
y
+
l
E
sin
ϑ
−
h
0
cos
ϑ
l
0
+
ϑ
1(2)
;
ISSN 0236-3941. HERALD of the BMSTU. Series “Mechanical Engineering”. 2014. No. 1 29