Numerical analysis of high density alloys and elongated projectiles’ velocity and strength effect on their penetration into a steel target

2 / 17

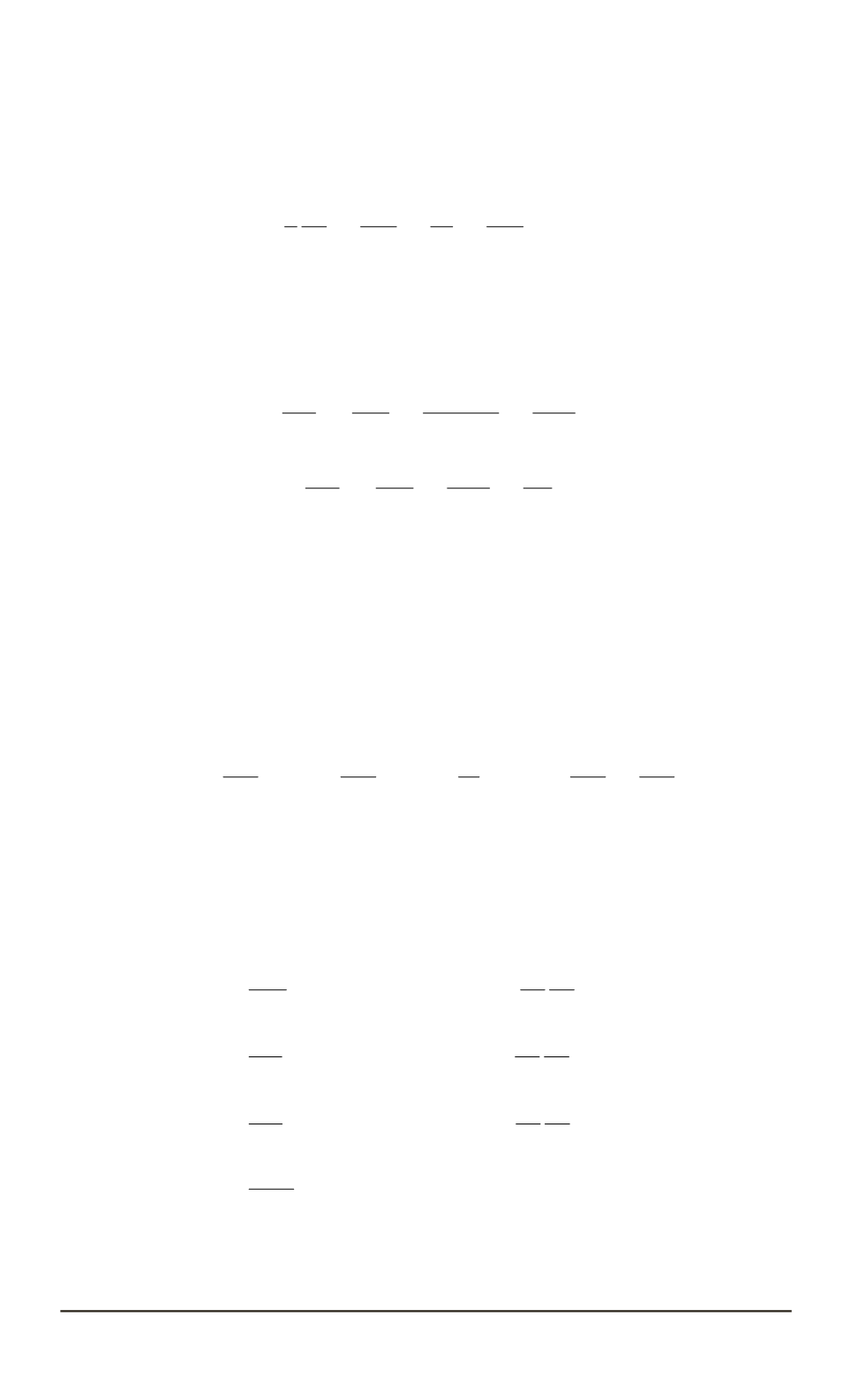
deformable medium (of target and projectile materials) can be described
by the following set of equations of the continuum mechanics [1].
Materials density ρ changes according to the law of mass conservation
in the notation of calculus (continuity equation):
1
ρ
dρ
dt
+
∂υr
∂r
+
υ r
r
+
∂υz
∂z
= 0
here υ r, υz are radial and axial components of the material particle velocity.
Radial and axial accelerations of the material particles are defined
according to the impulse law in the notation of calculus:
ρ
dvr
dt
=
∂σr
∂r
+
σr − σθ
r
+
∂τrz
∂z
;
ρ
dvz
dt
=
∂σz
∂z
+
∂τrz
∂r
+
τrz
r
,
here σr, σz , σθ τrz are normal and tangential components of the stress
tensor.
Mechanical stresses due to the deformation of the target and projectile
materials (metals) are calculated according to the model of the compressible
elastoplastic medium. Strain rate tensor components ˙ εr, ˙ ε z , ˙ εrz , of the
materials are expressed by velocity vector components derived from
kinematic equations:
˙ εr =
∂vr
∂r
; ˙εz =
∂vz
∂z
; ˙εθ =
vr
r
; ˙εrz =
∂vz
∂r
+
∂vr
∂z
.
Tension evolution of the materials which can undergo significant plastic
deformations here is based on the incremental plasticity theory [1]. In this
case the major equations of this theory (Prandtl —Reiss equations) are
written in the form of the following differential equations:
d sz
d t
+ 2G ˙
λsz = 2G ˙ εz +
1
3ρ
dρ
dt
;
dsr
dt
+ 2G ˙
λsr = 2G ˙ εr +
1
3ρ
dρ
dt
;
dsθ
dt
+ 2G ˙
λsθ = 2G ˙ εθ +
1
3ρ
dρ
dt
;
d τrz
dt
+ 2G ˙
λτrz = G ˙ εrz ,
here sz , sr, sθ are normal components of the stress tensor deviator, G is a
medium shear modulus, ˙
λ is scalar factor defined by a specific capacity of
66 ISSN 0236-3941. HERALD of the BMSTU Series “Mechanical Engineering”. 2015. No. 1