two-component injectors are used), film coolant flow-rate, cooling system
design, film coolant flow-rate in the periphery injectors, etc. The main
criteria for selecting the film cooling parameters are thermal and energy
efficiency. Thermal efficiency is defined as an ability of the film to prevent
the combustion chamber wall from heating above the maximum operating
temperature of material. Energy efficiency is defined as minimizing specific
impulse losses due to film cooling. These two criteria are contradictory. The
paper analyzes the factors affecting the thermal efficiency.
In [4] the thermal efficiency of film cooling at subsonic velocities of
both the film and the main flow is determined as follows
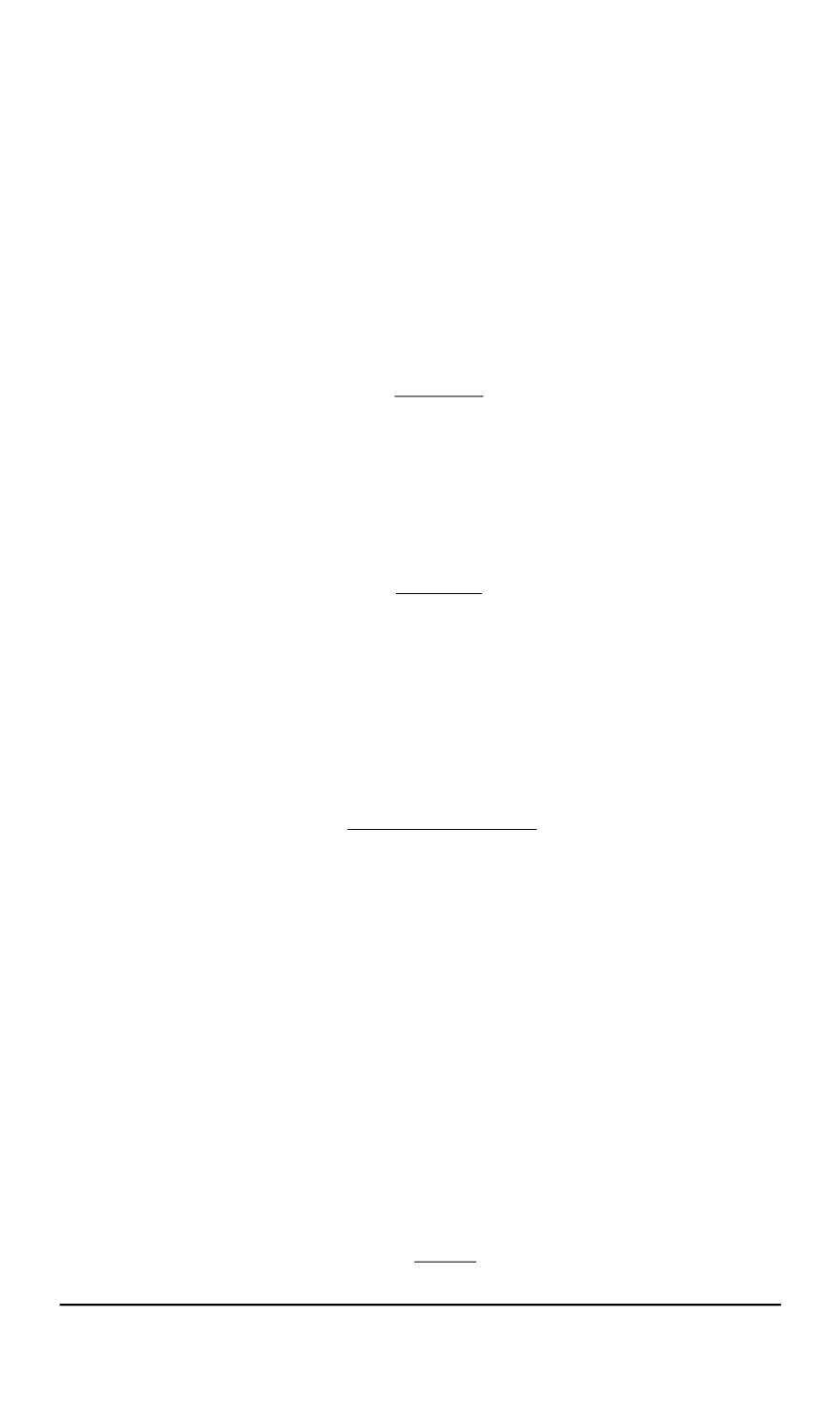
η
=
T
w
−
T
∞
T
f
−
T
∞
,
(1)
where
T
w
is the wall temperature gas side;
T
∞
is the main flow thermodynamic
temperature;
T
f
is the film gas temperature.
In [5] the adiabatic efficiency of film cooling is determined according
to the following criterion:
η
=
T
0
−
T
ad
T
0
−
T
f
,
(2)
where
T
0
is the main flow temperature on the boundary layer edge;
T
ad
is
the adiabatic wall temperature.
Ambiguity of
T
0
,
T
∞
estimation as well as an assumption of the wall
adiabaticity make it impossible to use these criteria for estimating the LTRE
film cooling efficiency. Due to these reasons, the approach suggested in [6]
is more suitable:
θ
=
T
w.wof
(
x
)
−
T
w.f
(
x
)
T
w.wof
(
x
)
−
T
f
,
(3)
where
T
w.wof
(
x
)
and
T
w.f
(
x
)
are the wall temperatures without and with film
cooling;
x
is the current coordinate.
Further in this paper this criterion is used for estimating the film cooling
thermal efficiency.
Gas films have become the subject of numerous experimental and
theoretical studies both in Russia and abroad.
For instance, the paper [6] considers the efficiency of film cooling in a
rocket engine (RE) working on H
2
(g) + O
2
(l) mixture. Gaseous hydrogen
was used as a coolant.
The paper discusses the influence of the following factors on the film
cooling efficiency:
1) relative mass loading of the injection and main flows
M
determined
by the relation
M
=
ρ
2
u
2
ρ
∞
u
∞
,
(4)
ISSN 0236-3941. HERALD of the BMSTU. Series “Mechanical Engineering”. 2014. No. 1 81