Analysis of gas-dynamic processes and development of model of flows in hypersonic shock tube

8 / 21

part of the numerical solution permits to acheve the seventh order of
accuracy:
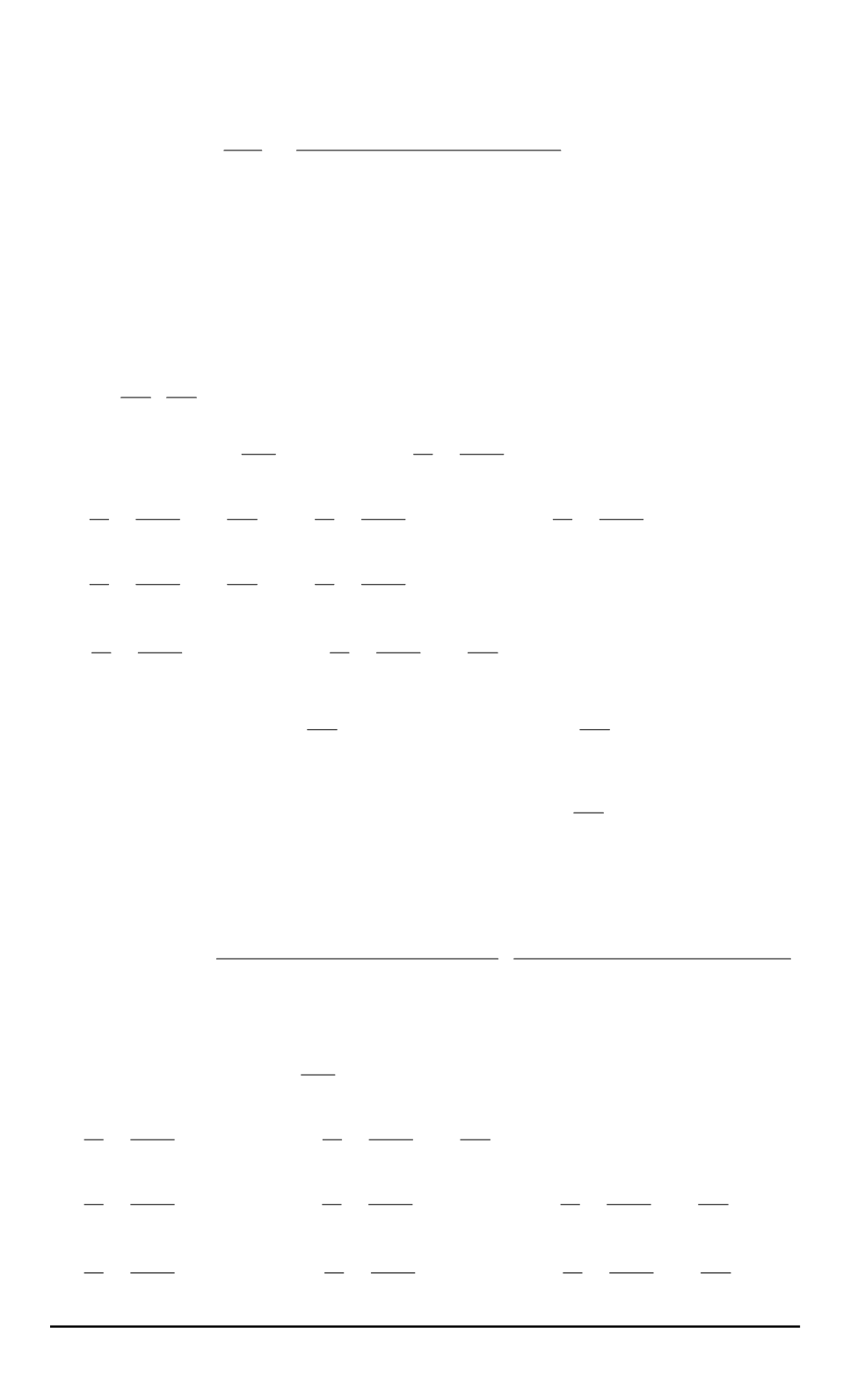
∂ ~Ui
∂t
+
F ~Ui+1 /2 − F ~U i−1/2
Δξ
= ~F
2.
Gas-dynamic parameters U n+1
i , U n
i are related to the centers of design
meshes, while the flows F n
i±1/2, Gn
i are to be determined on the surface
of these meshes. To rise the order of the approximation of the difference
scheme, one should retrieve the gas-dynamics parameters Y R,L
i±1/2, Y R,L
i on
the right (index R ) and on the left (index L) from the boundaries of
the design meshes. Then any function being retrieved Y (x) , [x = { ξ} ],
ξ ∈ [−
Δξ
2
,
Δξ
2
], can be represented by piecewise-polynomial distributions:
Y (ξ) = Yi +
∂Y
∂ξ i
[ξ − ξi] +
1
2!
∂2Y
∂ξ 2
i
[ξ − ξi ]2 −
−
2
3!
∂2Y
∂ξ 2
i
Δξ
2
3
+
1
3!
∂3Y
∂ξ3
i
[ξ − ξi] 3 +
1
4!
∂4Y
∂ξ 4
i
[ ξ − ξi ]4 −
−
2
5!
∂4Y
∂ξ 4
i
Δξ
2
5
+
1
5!
∂5Y
∂ξ5
i
[ξ − ξi] 5 +
+
1
6!
∂6Y
∂ξ 6
i
[ξ − ξi]6 −
2
7!
∂ 4Y
∂ξ4
i
Δξ
2
7) ,
where Y R
i+1 /2 = Y ξ =
Δξ
2
, Y L
i−1/2 = Y ξ = −
Δξ
2
, etc. Note, that
the formula data satisfy the balance relations: Yi =
1
Δξ
ξi+1/2 Z
ξi−1/2
(ξ) dξ.
These piecewise-polynomial distributions should be limited (to bring
them to the monotonous form) by a function-limiter ϕ (Y ) [6]:
ϕ (Yi )=min 1 , |Yi − max (Y k)|
Yi − max Yk−1/2, Yk+1 /2
, |Yi − min (Yk )|
Yi − min Yk−1/2, Yk+1/2 !,
where k = i − 2 , i − 1, i + 1, i + 2; i.e.,
Y (ξ) = Yi + ϕ (Yi)
∂Y
∂ξ i
[ξ − ξi ] +
+
1
2!
∂2Y
∂ξ2
i
[ξ − ξi] 2 −
2
3!
∂2Y
∂ξ 2
i
Δξ
2
3
+
+
1
3!
∂3Y
∂ξ3
i
[ξ − ξi] 3 +
1
4!
∂4Y
∂ξ 4
i
[ξ − ξi ]4 −
2
5!
∂4Y
∂ξ4
i
Δξ
2
5
+
+
1
5!
∂5Y
∂ξ5
i
[ξ − ξi] 5 +
1
6!
∂6Y
∂ξ 6
i
[ξ − ξi ]6 −
2
7!
∂4Y
∂ξ4
i
Δξ
2
7) .
10 ISSN 0236-3941. HERALD of the BMSTU. Series “Mechanical Engineering”. 2014. No. 1